Lever Rule: Screencast
Use a mass balance to derive the lever rule, which determines the amounts of liquid and vapor in equilibrium, given the overall mole fraction and the mole fractions in each phase for a binary mixture.
We suggest you list the important points in this screencast as a way to increase retention.
Another useful resource is the Lever Rule Wikipedia page.
Important Equations:
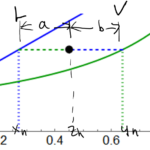
The lever rule for a binary system with vapor and liquid in equilibrium:
\[aL = bV\]
where \(a\) and \(b\) are the distances in the figure above and \(L\) and \(V\) are the number of moles of liquid and vapor, respectively.
The lever rule can also be written as:
\[(z_h – x_h)L = (y_h -z_h)V\]
where\(x_h\) and \(y_h\) are the liquid and vapor fractions, respectively, and \(z_h\) is the overall mole fraction of the mixture.

